Maths Complex analysis

Course Description:

Complex Analysis is a fundamental tool with numerous practical applications for solving physical problems. This course focuses on complex analytic functions—functions that possess a complex derivative. In contrast to calculus with real variables, the existence of a complex derivative imposes significant constraints on the function's properties. Applications covered in this course include harmonic functions, efficient techniques for evaluating difficult integrals, power series, and residue theory.

General Objectives:

  1. Provide students with a comprehensive understanding of complex analysis and its foundational concepts.
  2. Introduce students to complex functions, their derivatives, and methods for visualizing their graphical representations.
  3. Introduce students to advanced techniques for evaluating integrals, utilizing power series, and applying residue theory.
Target Audience:  

         3rd-year students in Microelectronic IC Design.

Circuit theory

Combinational and Sequential Logic

General Description:

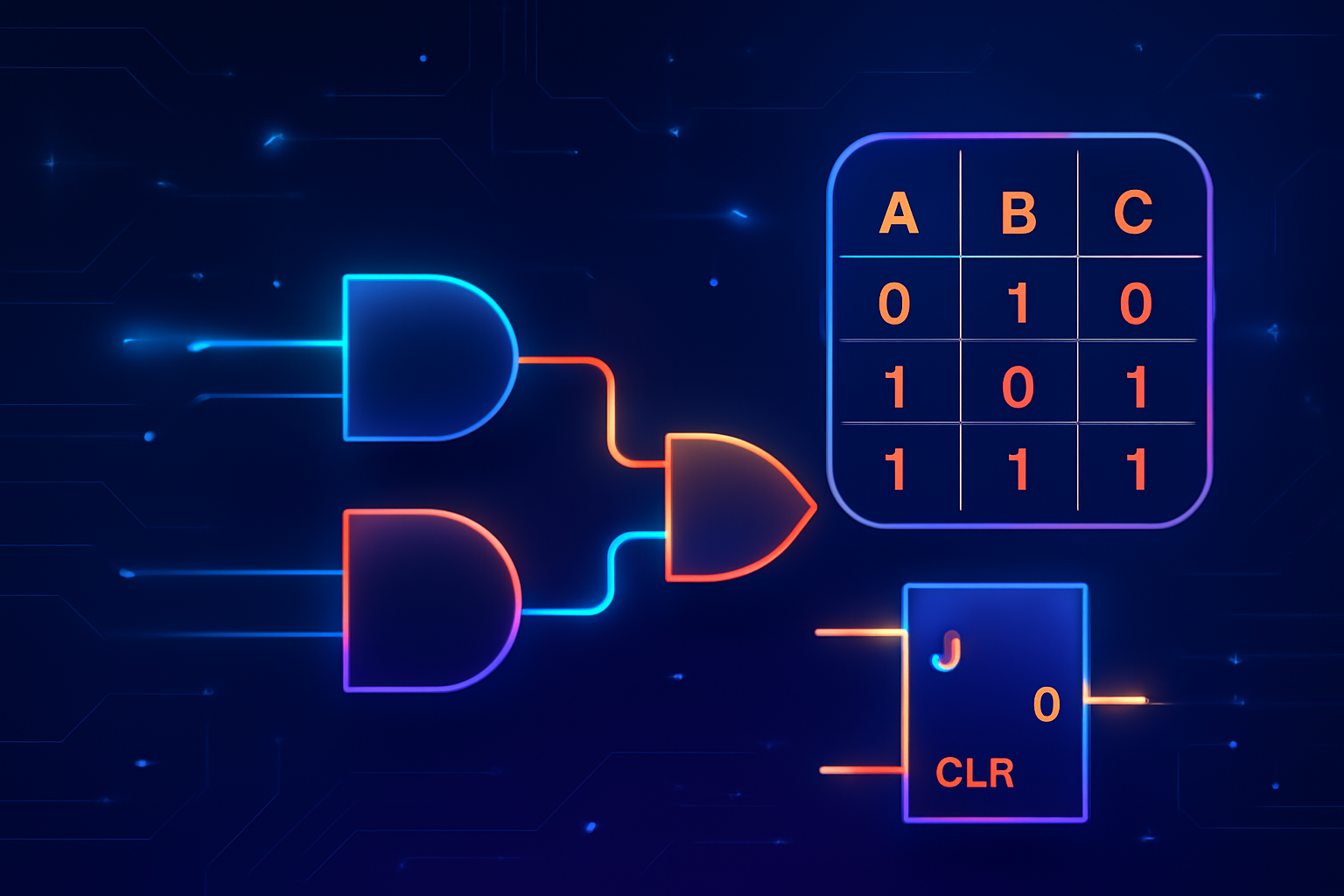
This course introduces students to the fundamental principles of digital logic, distinguishing between combinational and sequential logic. It enables them to model, design, and analyze logic circuits used in modern electronic systems (such as automatons, microprocessors, etc.).

Learning Objectives:

By the end of the course, students will be able to:

  • Understand the basic concepts of combinational and sequential logic.

  • Design simple logic circuits from Boolean expressions or truth tables.

  • Analyze the behavior of existing digital circuits.

  • Use flip-flops, registers, and counters in the design of sequential circuits.

  • Apply logical simplification tools (Boolean theorems, Karnaugh maps).

  • Model simple digital systems.

Course Content:

  1. Introduction to digital logic

  2. Boolean algebra and simplification of logic expressions

  3. Basic combinational circuits: logic gates, multiplexers, decoders, comparators, etc.

  4. Truth tables and logic diagrams

  5. Simplification methods: Karnaugh maps

  6. Arithmetic circuits: adders, subtractors

  7. Introduction to sequential logic: flip-flops (RS, D, JK, T)

  8. Design of sequential circuits: registers, counters,

  9. Practical applications and synthesis exercises

Signal Theory

Semiconductor Physics 1

Renewable Energy

Semester: 1
Teaching Unit: UET 3.1.1
Code: ER-T311
Credits: 2
Total Hours: 45 hours

Course Overview

This course provides an in-depth understanding of renewable energy systems, emphasizing sustainable technologies for energy production. Students will explore various renewable energy sources, their operating principles, and practical applications. By the end of the course, students will have gained critical insights into technologies such as solar energy, wind power, geothermal energy, and biomass.

Course Learning Objectives

- Understand the fundamental principles of renewable energy systems.
- Evaluate the environmental and economic impacts of various renewable energy technologies.
- Analyze the technological components of renewable systems, focusing on their design and efficiency.
- Compare renewable energy systems with traditional non-renewable energy sources in terms of sustainability and scalability.

Course Outline

1. Energy Sources

2. Low-Temperature Solar Thermal Energy

3. High-Temperature Solar Thermal Energy

4. Photovoltaic Solar Energy

5. Wind Energy

6. Biomass Energy

7. Geothermal Energy

8. Other Renewable Sources

Assessment

Final Examination: 100%

Bibliography

  • M. Kanoglu, Y. A. Cengel, J. M. Cimbala. Fundamentals and Applications of Renewable Energy, McGraw Hill Education, 2020.
  • J. A. Duffie, W. A. Beckman, N. Blair. Solar Engineering of Thermal Processes: Photovoltaics and Wind, John Wiley & Sons, 2020.
  • Erik Dahlquist. Biomass as Energy Source: Resources, Systems, and Applications, CRC Press, 2013.
  • J. Lemale. Geothermal Energy, Dunod, 2015.